Just like someone accelerating in empty space, an observer hovering above the horizon of a black hole would find herself surrounded by a warm sea of particles that are randomized since their entangled partners lie on the other side of the horizon. The information associated with the hidden particles would correspond to an entropy proportional to the area of the horizon. However, unlike an accelerating observer in empty space, the horizon, the temperature, and the entropy of the black hole would be objective phenomena that all observers outside of the horizon would agree on.

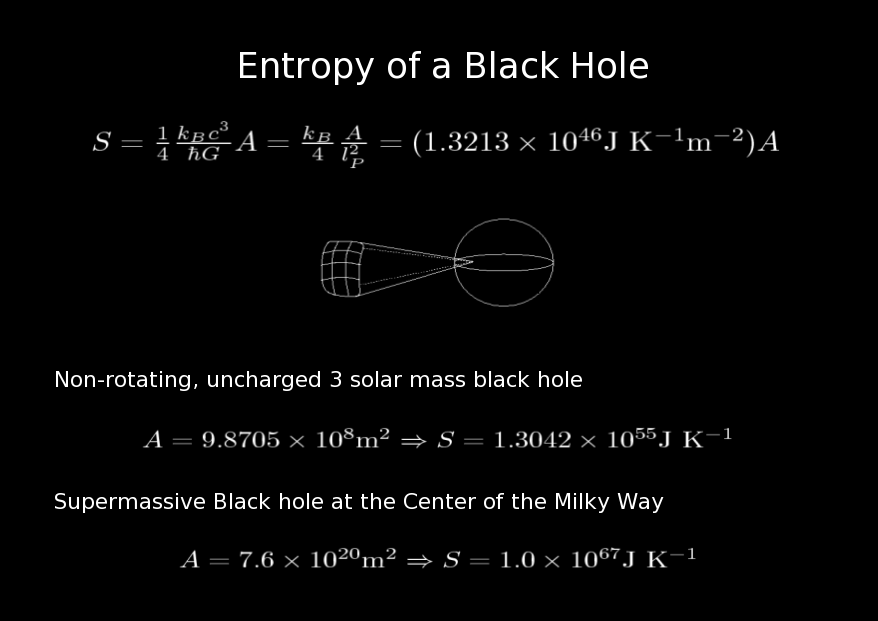
I have written the equation for the entropy S above. The entropy is the Boltzmann constant times the area of the horizon measured in units of 4 times the Planck area.

The smallest stellar black holes have masses of around 3 times the mass of our sun. The Schwarzschild radius for a black hole of this mass is about 9 km, which means that the area of the horizon of a non-rotating black hole of this size would be approximately 10⁹ m². Therefore, a non-rotating black hole of this size would have an entropy of around 1.3 × 10⁵⁵ J K^-1. The supermassive black hole at the center of our galaxy has a mass of about 2.6 million solar masses and a Schwarzschild radius of approximately 7.8 million km. This means that its horizon would have an area of about 7.6 × 10²⁰ m² and it would have an entropy of approximately 1.0 × 10⁶⁷ J K^-1 if it wasn't charged or rotating.

The horizon of a black hole is like a computer screen where each 4 Planck units of area corresponds to a pixel that codes for a bit of information. Notice that the black holes above contain huge amounts of information.