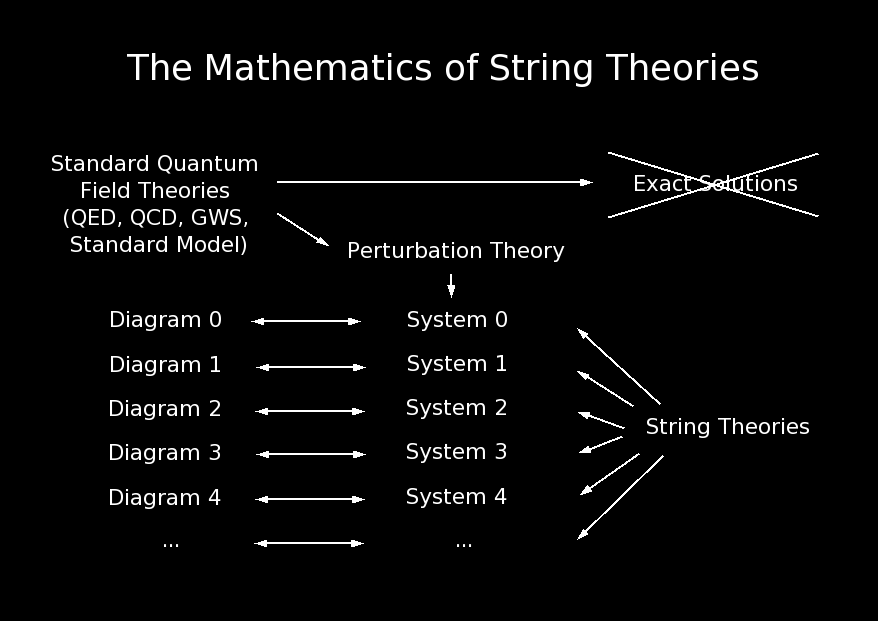
The story of modern string theories starts from the standard quantum field theories. Quantum field theories are quantum mechanical theories derived in the context of the flat spacetime of special relativity. The standard quantum field theories describe three of the four fundamental forces in nature. Quantum electrodynamics (QED) describes electromagnetism, quantum chromodynamics (QCD) describes the strong force that results from the interaction of quarks, antiquarks, and gluons in hadrons and mesons, and the Glashow-Weinberg-Salam theory (GWS) is a unified description of the weak nuclear force and electromagnetism. Their synthesis, which describes all three of these forces, is called the Standard Model.

No one knows how to find exact solutions to the equations of the standard quantum field theories or even show that they're mathematically consistent. Instead, physicists apply an approximation technique called perturbation theory to construct approximate solutions to the theories. These approximations have matched the results of all experiments performed so far to an amazing degree of accuracy, to 11 decimal places in the case of QED. However, no one knows whether the assumptions used in constructing the approximations are warranted or if these approximations are consistent with true solutions to the theories.

Applying perturbation theory means assuming that some processes that a theory represents are less important than others and constructing a sequence of approximate sets of equations that include increasingly small influences from the weaker processes. Ideally, it is possible to solve at least the most important approximate sets of equations and thereby construct reasonably accurate approximate solutions to the original theory.

Richard Feynman came up with an interesting way to look at the sets of approximate equations. When modeling an interaction between particles, he suggested that each set corresponds to a different way that the interaction might take place and that the true solution, which determines what takes place in the interaction, is simply the sum of all of the different ways that the interaction might take place, where each particular way is weighted by the probability of that way occurring. Each different way that an interaction might take place is represented by a Feynman diagram.

One shortcoming of the perturbation solutions and presumably of the exact theories is that attempts to calculate certain fundamental quantities, such as the charge of the electron, result in infinite answers. The infinities come from processes that involve high energy virtual particles, which are allowed to exist for short times due to the uncertainty principle. In some theories, including the standard quantum field theories, only a small number of quantities are infinite and the theory can be rescued by replacing the infinite quantities with their known finite values. After this is done, the theory produces reasonable and very accurate values for any other desired quantities. Rescuing a theory in this way is called "renormalizing" the theory and theories where this can be done are called "renormalizable".

As an aside, I'll mention that most theories, including general relativity, are not renormalizable. In general relativity, higher energy particles have larger gravitational effects, which causes the perturbation series to diverge. In the standard quantum field theories, higher energy interactions have less influence, which makes renormalization possible.

Another way of eliminating the pesky infinities from quantum field theories is to assume that the basic participants in fundamental processes are strings rather than particles. This is not done within the exact equations of the theories but is done within the sets of perturbation equations instead. Both the exact quantum field theories and the sets of perturbation equations are background-dependent. So any string theories created in this way are background-dependent too.